Note: This is the first of a many-part blog series addressing common student weaknesses on the SAT. Each post will be accompanied by a YouTube video from our Senior Director of Instruction, Dr. Megan Stubbendeck.
In the first edition of our SAT student weakness blog series, we focus our attention on one of the most commonly tested parts of the Math section: linear equations. On the SAT, questions about lines can cover any difficulty level, from straightforward questions about slopes and intercepts to complicated questions that require us to apply linear functions to real-world problems. If you’re unsure of how to find the equation of a line, or you think you might need a refresher on the basics, check out this helpful video from Megan:
Once you’re comfortable with the ideas and techniques that Megan described above, let’s jump into a more difficult application of linear equations: solving for multiple variables in a multi-equation system. First, let’s check out this sample SAT question:

This is a very common question type in which we are given the equations of two lines and are asked which coordinate pair satisfies both equations. If you see a question like this on the SAT, there are two equally valid techniques that will get you the correct solution. We’ll look at both, and you can choose which one to use on future questions.
The first technique is the substitution technique. Here, we pick one equation and solve for a variable. I’m going to take the second equation and solve for y.
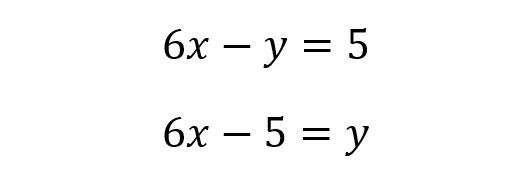
If you’ve watched Megan’s video, you should recognize this as the equation of a line in slope-intercept formula. (Quiz yourself: what are the slope and y-intercept of this line?)
Next, we go back to the first equation, and wherever we see “y”, we substitute “6x – 5”. This gives us one equation in one variable:

We’re almost there. To find y, we simply plug x=2 back in to either of the two equations:
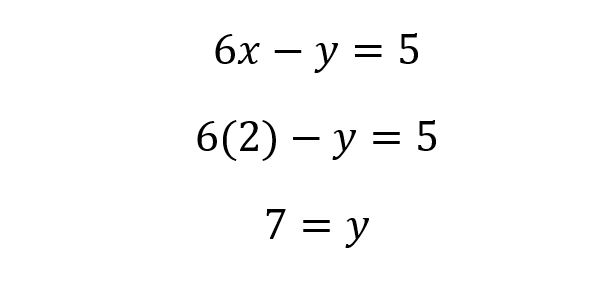
With x being equal to 2 and y being equal to 7, our answer is (2, 7), or (B).
The second technique for solving these problems is the elimination method. The goal of the elimination method is the same as that of the substitution method: to manipulate the equations so that we have one equation in one variable. But the elimination method does this in a much different way. Take a look at the original two equations from the problem. Notice that the coefficient in front of the two x terms is the same: six. In this case, we can subtract the entire second equation from the first equation, eliminating the x terms:
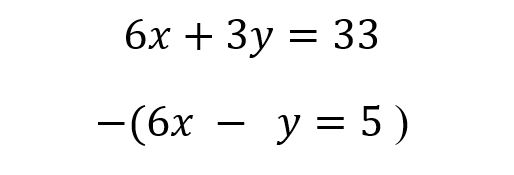
Next, we simply subtract each term from the second equation from the first. 6x — 6x = 0, so the x terms are eliminated. 3y — (–y) = 4y, and 33 — 5 = 28.
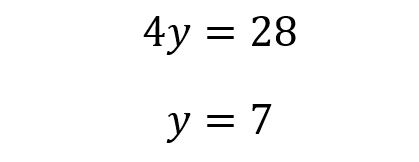
The final step is the same as in the substitution method. We choose either equation and substitute y = 7, again finding that our correct answer is (2, 7).
If you’ve mastered the basics in Megan’s video, you’ll be able to apply them to a variety of problems. When you’re asked to find the coordinate pair that satisfies both equations, use whichever technique—substitution or elimination—you’re more comfortable with.
Later in the week, we’ll be back with another blog and video addressing a common student weakness in the SAT Math section!

